Vettore P invariante minimale

Cosa sono i vettori P-invarianti

I vettori P-Invarianti sono detti minimali se non esiste un altro vettore minimale x' tale che $$ x' < x $$

A cosa servono i vettori p-invarianti minimali?

Ogni vettore P-invariante non minimale può essere ottenuto come combinazione lineare con coefficienti interi di uno o più vettori P-invarianti minimali.

Nota. Un vettore P-invariante minimale può essere di supporto minimo oppure no.

Per trovare gli eventuali vettori P-invarianti minimali posso usare un apposito algoritmo.

Un esempio pratico

Prendo in considerazione una rete marcata con tre posti e due transizioni

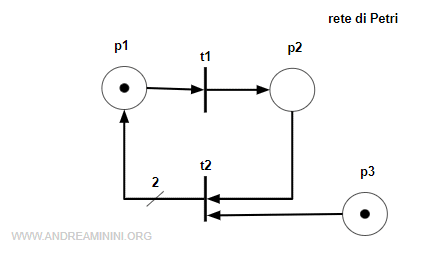
Calcolo la matrice di incidenza C della rete per differenza tra le matrici Post e Pre transizione.

$$ C =\begin{pmatrix} 0 & 2 \\ 1 & 0 \\ 0 & 0 \end{pmatrix} - \begin{pmatrix} 1 & 0 \\ 0 & 1 \\ 0 & 1 \end{pmatrix} $$

$$ C =\begin{pmatrix} -1 & 2 \\ 1 & -1 \\ 0 & -1 \end{pmatrix} $$

Il vettore x = [1 1 1] è P-invariante perché

$$ x^T \cdot C = \begin{pmatrix} 1 \\ 1 \\ 1 \end{pmatrix}^T \cdot \begin{pmatrix} -1 & 2 \\ 1 & -1 \\ 0 & -1 \end{pmatrix} = [1 \: 1 \: 1] \cdot \begin{pmatrix} -1 & 2 \\ 1 & -1 \\ 0 & -1 \end{pmatrix} = [0 \: 0] $$

I supporti del P-vettore invariante sono $$ ||x|| = \{p_1,p_2,p_3\} $$

Un altro vettore P-invariante è x'=[2 2 2] ma non è minore di x=[1 1 1].

$$ x^T \cdot C = \begin{pmatrix} 2 \\ 2 \\ 2 \end{pmatrix}^T \cdot \begin{pmatrix} -1 & 2 \\ 1 & -1 \\ 0 & -1 \end{pmatrix} = [2 \: 2 \: 2] \cdot \begin{pmatrix} -1 & 2 \\ 1 & -1 \\ 0 & -1 \end{pmatrix} = [0 \: 0] $$

Poiché non esistono altri P-vettori invarianti minori di x, il vettore x=[1 1 1] è detto P-vettore invariante minimale.

Ad esempio, tutti i vettori non nulli minori di x non sono P-invarianti.

$$ [1,0,0] \cdot C = [-1, 2] \\ [0,1,0] \cdot C = [1, -1] \\ [0,0,1] \cdot C = [0, -1] \\ [1,1,0] \cdot C = [0, 1] \\ [1,0,1] \cdot C = [-1, 1] \\ [0,1,1] \cdot C = [1, -2] $$

Nota. Il vettore P-invariante x'=[3 3 3] posso scriverlo come combinazione lineare del vettore P-invariante minimale x=[1 1 1]. $$ x'= \begin{pmatrix} 3 \\ 3 \\ 3 \end{pmatrix} = a \cdot x $$ $$ x'= \begin{pmatrix} 3 \\ 3 \\ 3 \end{pmatrix} = a \cdot \begin{pmatrix} 1 \\ 1 \\ 1 \end{pmatrix} $$ con i coefficienti interi a=[3,3,3]^T.

E così via.